<
81st birthday
Back to birthday list
83rd birthday
>
Top 10 players on their 82nd birthday
|
# |
Player | Rating | +/- |
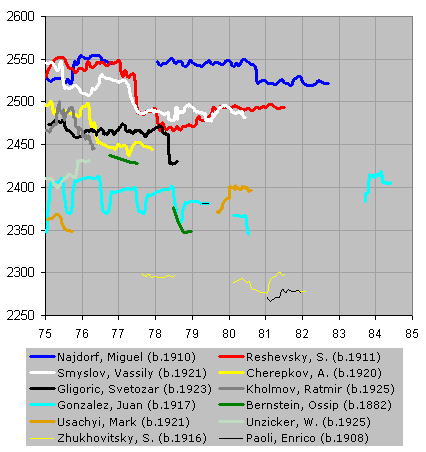
| 1 | Najdorf, Miguel | 2530 | 74 |
| 2 | Zhukhovitsky, Samuil M | 2278 | 73 |
| 3 | Paoli, Enrico | 2276 | 75 |
| 4 | Denker, Arnold S | 2253 | 67 |
| 5 | Wagman, Stuart | 2161 | 63 |
About these ratings
All players on their 82nd birthday
|
# |
Player | Rating | +/- | Best? | 75%? | Rating Date |
| 1 | Najdorf, Miguel | 2530 | 74 | 98.4% | #1 | 03/31/1992: #240 |
| 2 | Zhukhovitsky, Samuil M | 2278 | 73 | 0.7% | #4 | 11/30/1998: #2595 |
| 3 | Paoli, Enrico | 2276 | 75 | 0.7% | #4 | 12/31/1989: #1422 |
| 4 | Denker, Arnold S | 2253 | 67 | 0.2% | #4 | 01/31/1996: #2949 |
| 5 | Wagman, Stuart | 2161 | 63 | #5 | 05/14/2001: #3613 |
For each player on this list, the rating they possessed on their 82nd birthday is listed. That rating was the last one calculated while they were 81 years of age, so it was their "current" rating on the day they turned 82. Players who played
an insufficient number of games in recent years will not appear on the list, even though you may see them on lists for younger and/or older birthdays. Any players who had been completely inactive for three years are removed from the
list.
Definition of columns in the above list:
# refers to the player's all-time rank on this list, compared to all other players when they turned 82.
Player is the full name of the
player. Click on the player's name to see a career progression of
their ratings.
Rating is the calculated rating for each player on their 82nd birthday.
+/- is the standard deviation of the player's rating estimate. It is based on a geometric average of the number of rated games played in past years. Players who play very frequently will have a smaller +/- value, indicating a greater
confidence in the accuracy of their rating. Players whose +/- value exceeds 76 are classified as "inactive" and removed from the ranking list due to their infrequent play against rated opponents. Mathematically, a player's rating is an estimate
of their exact strength on their 82nd birthday. The
+/- value represents the standard deviation of that estimate; the player's true strength on their 82nd birthday should
be within one standard deviation of their calculated rating approximately 68% of the time, and within two standard deviations of their calculated rating approximately 95% of the time.
Best? is the estimated likelihood that the player
was stronger on their 82nd birthday than anyone else turning 82. Since ratings are known to be inaccurate, it is possible that a lower-rated player was actually much stronger than their rating would indicate, and it is also possible
that other players were weaker than their ratings would indicate. This number
indicates the likelihood that the player was indeed the strongest player ever among players turning 82. On a particular list, it will add up to 100% across the complete list of all players. This value is only calculated for the top 100
players on any rating list.
75%? gives a 75%-confidence world ranking for the player. Because ratings are known to be inaccurate, even if a particular player had the third-highest rating ever, among players turning 82, we cannot say with 100%
certainty that they were truly one of the three
strongest 82-year-old players. This number indicates the all-time rank that we are 75% sure the player is "no worse than". Thus if a player has a value of #12 in this column, we are 75% sure that the player is one of the twelve strongest
players ever to turn 82. This value is only calculated for the top 100 players on any rating list.
Date is the exact date that the rating applied to. Click on that date to see the sorted list of all players' ratings
on that date.